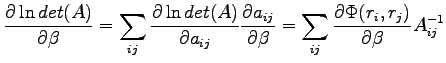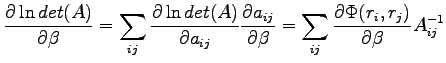The logarithmic derivatives

In pairing trial-function the variation of a parameter involves all terms of the matrix and so using D.3 the logarithmic derivatives will be:

$$\frac{\partial \ln det(A)}{\partial \beta} = \sum_{ij}\frac{\part... ...al \beta} = \sum_{ij} \frac{\partial \Phi(r_i,r_j)}{\partial \beta} A_{ij}^{-1}$$

If only the k-th orbital depends by 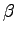$\beta$ we obtain:

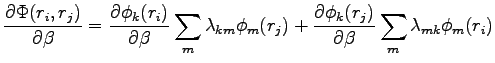$$\frac{\partial \Phi(r_i,r_j)}{\partial \beta} = \frac{\partial \p... ...r_j) + \frac{\partial \phi_k(r_j)}{\partial \beta}\sum_m\lambda_{mk}\phi_m(r_i)$$ (B.15)

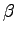If $\beta$ is one of the 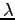$\lambda$ parameter the derivative will be:

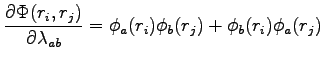$$\frac{\partial \Phi(r_i,r_j)}{\partial \lambda_{ab}} =\phi_a(r_i)\phi_b(r_j) + \phi_b(r_i)\phi_a(r_j)$$ (B.16)

because 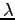$\lambda$ matrix is symmetric.