Gradients and Laplacian

Using the formula D.3 and the fact the only a column or a row may depends from a given electronic coordinate we obtain:

$$\nabla^2_i \ln \left\vert A \right\vert = \sum_{k} \nabla^2_i \Phi(r_i,r_k) A_{ik}^{-1}$$

$$\vec{\nabla}_i \ln \left\vert A \right\vert = \sum_{k} \vec{\nabla}_i \Phi(r_i,r_k) A_{ik}^{-1}$$ (B.12)

where:

$$\nabla^2_i \Phi(r_i,r_k) = \sum_{l,m}{\lambda_{l,m} \nabla^2_i \phi_{l}(r_i) \phi_{m}(r_j)}$$ (B.13)

$$\vec{\nabla}_i \Phi(r_i,r_k) = \sum_{l,m}{\lambda_{l,m} \vec{\nabla}_i \phi_{l}(r_i) \phi_{m}(r_j)}$$ (B.14)

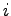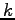for spin down we have to exchange $i$ with $k$ in all these equations.