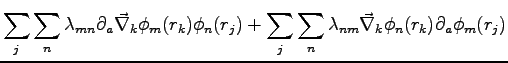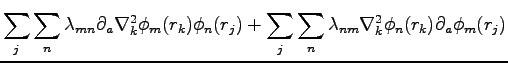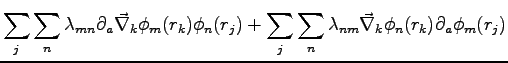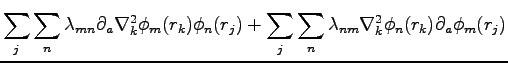Derivatives of the local energy

It is very simple to evaluate the terms appearing in B.5 and B.6 in fact using B.29 and B.28 and considering only the 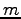$m$ orbital dependent by the parameter $a$ we obtain:

$$\partial_a \vec{\nabla}_k \ln U = \sum_{j}\sum_{n} \lambda_{mn} \partial_a \vec{\nabla}_k \phi_m(r_... ...\sum_{j}\sum_{n} \lambda_{nm} \vec{\nabla}_k \phi_n(r_k) \partial_a \phi_m(r_j)$$

$$\partial_a \nabla^2_k \ln U = \sum_{j}\sum_{n} \lambda_{mn} \partial_a \nabla^2_k \phi_m(r_k) \... ... + \sum_{j }\sum_{n} \lambda_{nm} \nabla^2_k \phi_n(r_k) \partial_a \phi_m(r_j)$$